Heavy fermion materials
,1 ,1 ,2 ,3 ,1 ,4 ,5 ,1 ,6 ,1 ,7 ,1 ,8 and 9
1Max-Planck-Institut für Chemische Physik fester Stoffe, 01187 Dresden, Germany 2University of California, Irvine, California 92697, USA 3HH Wills Laboratory, University of Bristol, Bristol BS8 1TH, UK 4Center for Correlated Matter, Zhejiang University, Hangzhou, Zhejiang 310058, China 5Institute of Physics, Goethe-Universität Frankfurt, 60438 Frankfurt/Main, Germany 6Dept. of Condensed Matter Physics, Weizmann Institute of Science, 76100 Rehovot, Israel 7Dept. of Physics and Astronomy, Rice University, Houston, TX 77005, USA 8Los Alamos National Laboratory, Los Alamos, NM 87545, USA 9Institut für Mathematische Physik, TU Braunschweig, 38106 Braunschweig, Germany
Electronic correlations give rise to a plethora of interesting phenomena and phases. For example, hybridization between 4f and conduction electrons in heavy fermion metals may result in the generation of low-energy scales that can induce quantum criticality and unconventional superconductivity (see Special Issue, Ref. 1). These complex interactions often operate, and compete, on different lengths scales. One of the most important techniques that helped shaping our understanding of nonlocal correlations, both magnetic and superconducting, has been scanning tunneling spectroscopy (STS) with its unique ability to give local, microscopic information that directly relates to the one-particle Green’s function. We complement these local measurements with global probes, most importantly magnetotransport (see review on Hall effect, Ref. 2), magnetization and x-ray diffraction measurements, in order to unambiguously assign the local, surface-sensitive findings by STS to bulk properties of the single crystals under scrutiny.
As a pivotal prerequisite for a possible disentanglement of the involved interactions, to rule out inadvertent influences of impurities as well as to successfully relate local with global properties, samples of highest possible quality need to be investigated. Here, the so-called 115 family of compounds (CeMIn5 with M = Co, Ir, Rh) exhibiting superconductivity, magnetism and Fermi surface changes, are a clear-cut example for which we could identify nanometer-scale defects, Wirth et al. 2014 [3]. A substitution series of these compounds was recently instrumental in identifying the Ce 4f wave function as a significant parameter for the realization of either a superconducting or an antiferromagnetic ground state, Willers et al. 2015 [4]. For CeCoIn5 a field-induced quantum critical point (QCP) of spin-density-wave (SDW) type is extrapolated to exist inside the superconducting phase. Single crystals of 115 compounds were provided through our long-term collaboration with groups led by Dr. Zachary Fisk (UC Irvine, USA) and Dr. Joe D. Thompson (Los Alamos National Laboratory, USA). Similarly, in CeCu2Si2 a SDW type of QCP was established in the vicinity of which superconductivity was demonstrated to be mediated by spin fluctuations, Steglich et al. 2013 [5].
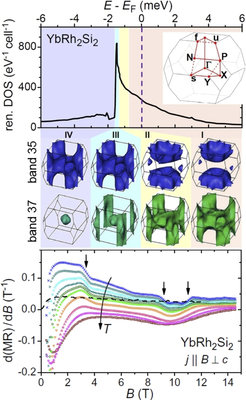
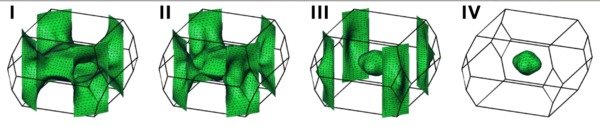
Very strong correlation effects can be realized through the above-mentioned hybridization between 4f and conduction electrons via the Kondo effect. In case of the prototypical heavy fermion metal YbRh2Si2 this can result in quasiparticle masses beyond a thousand times the free electron mass. Our low-temperature electronic transport measurements at high magnetic field (μ0H ≤ 15 T) in concert with thermal transport measurements (conducted in the group Novel states of matter on the border of magnetism) and renormalized band structure calculations by Prof. Gertrud Zwicknagl indicate that the de-renormalization of the quasiparticles, i.e. the destruction of the local Kondo singlets, occurs smoothly and likely requires magnetic fields of the order of 30 T to complete, Naren et al. 2013 [6], Pfau et al. 2013 [7]. On the other hand, the spin splitting of the very flat, hybridized bands results in three successive Lifshitz transitions which are confined to relatively narrow magnetic field intervals and clearly visible in magnetotransport [6] (see Fig. 1, lower panel) and thermopower measurement [7].