Quantum Tricritical Points
The concept of 'critical point' is a very old one and it is defined in thermodynamics as the end point of a line of 1st order phase transitions (phase equilibrium curve). A famous example is the critical point of water at which a continuous or 2nd order phase transition separates the liquid phase from the gaseous phase and large thermal fluctuations can be observed. These fluctuations are responsible for what is known as critical opalescence.
The concept of 'quantum critical point' (QCP), on the other hand, is a rather modern one and it is nowadays strongly investigated mainly because of its importance in connection with unconventional superconductivity (see e.g. press release, 10 Juli 2017). The thermodynamic definition and meaning of this point is similar to that of the classical critical point, but not exactly the same: the QCP is the zero temperature point of a line of 2nd order phase transitions. Here, not thermal but quantum fluctuations play the most important role.
QCPs are generally studied in antiferromagnetic (AFM) metals and, indeed, unconventional superconductivity was indeed often found at and near such QCPs. In these systems, the 2nd order phase transition line is the pressure evolution of the AFM ordering temperature. Yet, quantum critical points are extremely rare in ferromagnetic (FM) metals (see, e.g., M. Brando, D. Belitz, F. M. Grosche, and T. R. Kirkpatrick, Metallic quantum ferromagnets, Rev. Mod. Phys. 88, 025006 (2016)) and superconductivity was only found in a few U-based compounds. Recent experimental and theoretical works have shown that, under particular conditions, some FM metals change their magnetic ordering from FM to AFM just before reaching the QCP under pressure. This situation allows for the possibility of having multiple critical points and therefore, at zero temperature, quantum multicritical points.
One of these materials is the metallic magnet NbFe2, a relatively simple binary compound but with the peculiar characteristic of showing a change from a low-temperature FM state in an AFM state under small pressure. If a magnetic field is applied along a particular crystallographic direction while the system is in the AFM state, a 1st order phase transition into the paramagnetic state can be induced (red line in Fig.1). In this material, however, this line is separated from a 2nd order phase transition line (blue line in Fig. 1) by a point (red point in Fig. 1) that, per definition, is a tricritical point (TP). In our work, in collaboration with international colleagues of the universities of Bristol (press release of the University of Bristol), Munich and Cambridge, we have followed and investigated the properties of the TP to zero temperature - a quantum tricritical point (QTP) - in a real material for the first time. We successfully used a well-known two-order-parameter Landau theory to understand the phase diagram of NbFe2, which is considered to be generic for all systems with avoided FM QCP through an adjacent AFM phase like in CeRuPO or in the prominent heavy-fermion compound YbRh2Si2.
Our experimental contribution to this work was mainly oriented towards the detection of thermodynamic signatures of the magnetic 1st and 2nd order phase transitions in NbFe2. The weakness of these phase transitions has made this a particularly complicated matter. For this reason, we used an extremely sensitive dilatometer (inset of Fig. 1), developed in our institute over the past years. Meanwhile it can be purchased from a spin-off company set up by Robert Kuechler: http://www.dilatometer.info/.
MB ; RK / CPfS
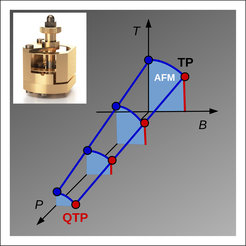
1. Generic phase diagram of an AFM system in which a 1st order phase transition (red line) can be induced by a magnetic field (B). The tricritical point (TP) separates a line of 2nd order phase transitions (blue line) from a line of 1st order phase transitions. Pressure (P) can tune this TP to zero temperature which ends at the quantum tricritical point. Insert: Dilatometer used in this work: A sample is clamped between the two plates of a capacitor whose capacitance changes when the sample expands or contracts. This change in capacitance can be measured very accurately. This dilatometer enables to measure at temperatures between 300 to 0.02 K changes in length of ΔL ≈ 10-11 - 10-12 m which are about hundred times smaller than the separations of the atoms in the crystal lattice.
