Heusler Theory - S. Chadov
Materials design implies a consistent interaction between theory and experiment. In collaboration with different experimental groups we provide the first-principle description, explanation and modeling of materials characteristics. Our basic area is the family of ternary Heusler compounds, which due to their rich chemical variability, solves a large number of technological tasks. There are several simple rules, concerning chemical and magnetic ordering which hold rather unambiguously in most of the known Heusler systems and allow for the straightforward modeling of their properties.
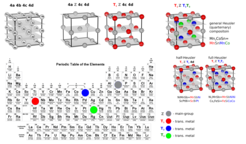
Chemical and magnetic ordering in Heusler compounds. Most of the Heusler compounds exhibit fcc cubic structure. In some cases (as e.g. Mn3Ga) it becomes unstable and distorts by typically elongating or suppressing along the [001]-direction (tetragonal c-axis). However, in any of these structures the system obeys the same chemical ordering. It is illustrated in FIG.1. For simplicity, we start from an empty fcc lattice to which we attribute the space group F-43m (216). This provides four Wyckoff sites: 4a (0,0,0), 4b (½,½,½), 4c (¼,¼,¼) and 4d (¾,¾,¾). First step is arbitrary: we fill in one of them, say 4b, with a main-group element Z. The next step is the most important: we fill the sublattice 4a (within the same atomic layer with 4b) with an earliest (located leftmost in the periodic table) transition element T1 (marked by red). This results into T1Z NaCl-structure which is "prior" substructure in the ordering hierarchy. The rest steps are simple: we just fill in the remaining Wyckoff positions (4c/4d) with the later (located on the right from T1 in the periodic table) transition elements (T2 and T3, marked blue and green). Following the 4a4b4c4d sequence, the general quarternary Heusler structure is written as T1ZT2T3. In particular, NiMnSb is notated as MnSbNiVc, with Vc (in 4c or 4d) being a void, which formally behaves as a late transition element. This type of systems is often called "half-Heuslers". In case of T2=T3 (e.g. Co2MnSi) we obtain the so-called "full-Heusler" type. In this case, an additional symmery operation, namely the inversion, unites 4c and 4d classes into a single 8c class by lifting the space group index up to 225 (Fm-3m). On the other hand, the Heusler compound of Mn2CoGa composition will exhibit MnGaMnCo (but not CoGaMnMn) ordering, in which two Mn atoms are nonequivalent due to their different 4a and 4c Wyckoff positions. These structure types are often called "inverse". With no restriction the same rules are applied to the tetragonally-distorted structures. In full analogy with the cubic systems, the space group of a general (noncentrosymmetric) tetragonal Heusler structure T1ZT2T3 is I-4/m2 (119) assuming four Wyckoff positions: 2a (0,0,0), 2b (0,0,½), 2c (½,0,¼) and 2d (½,0,¾). In case T2=T3 (inversion is added), last two classes are united into a single 4c (½,0,¼) class and the point group is lifted up to I4/mmm (139).
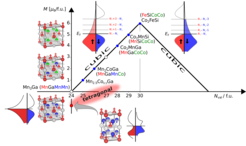
Most of the cubic Heusler compounds obey the famous Slater-Pauling rule stating that their magnetization M changes linearly with a number of valence electrons Nval, exactly by 1 μB per electron in a formula unit: M [μB/f.u.] = Nval - 24. Such behavior is a direct consequence of the so-called half-metallicity meaning that one of the spin-channels (normally the minority-spin, left side of the Slater-Pauling line) exhibits a semiconducting band gap at EF (see FIG.2). Such compositions as Co2FeSi or Co2MnGa with high M values (of 6 and 5 μB/f.u.) are full ferromagnets, with a parallel orientation of all magnetic sublattices. The situation changes for Mn-rich Heusler systems, e.g. Mn2CoGa, with a lower magnetization (2 μB/f.u.) but high local moments on Mn atoms (~3-4 μB/f.u.): such systems represent a class of ferrimagnets. Thus, within the "inverse" (cubic or tetragonal) Heusler arrangement, Mn magnetic moments on 4a and 8c (or 4c/4d) will couple to one another antiparallel. A peculiar type of a ferrimagnet, namely the fully-compensated ferrimagnet in cubic Heusler family is suggested by the Slater-Pauling rule for Mn3Ga (Nval=24). In this system the magnetization of Mn(4a) sublattice is fully compensated by Mn(8c) one, remaining a half-metal at the same time (!). In reality, due to the electronic instability in the spin-up channel, the system undergoes a tetragonal distortion and the half-metallicity is lost together with the magnetic compensation. The M(Nval) trend for the tetragonal Heusler systems is shown in FIG.2 (red blurred area with a weaker average slope as compared to the cubic subgroup). In general, it is worth to admit the following three points in the outlined trend: Nval=30 corresponding to the highest value of magnetization of 6 μB/f.u. in cubic Co2FeSi, Nval=27, where occurs the rearrangement from ferro- to ferri-magnetic order (e.g., noncollinear tetragonal Mn2RhSn, Mn2PtIn and Mn2IrSn ferrimagnets) and Nval=24 - the fully compensated ferrimagnet (e.g. cubic Mn1.5V0.5FeAl).
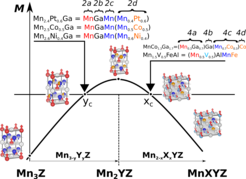
Mn-rich Heusler compounds. The rules discussed above can be used for a preliminary compositional engineering of the magnetic properties without any first-principles calculations. FIG.3 demonstrates the basic trend of the magnetization M in Mn-rich Heusler materials, describing the formation of two types of compensated ferrimagnets. Our starting point is Mn3Z (e.g., Mn3Ga tetragonal ferrimagnet) with a negative magnetization M (with respect to the orientation of magnetic moment on Mn(2a)). By substituting Mn with a late transition element Y on 4c (or 2c/2d), M approaches zero. At certain critical concentration (yc) it crosses a compensation point (some of the known compensated compositions, e.g. Mn2.4Pt0.6Ga are listed explicitly in FIG.3) and continues to grow in the positive direction. The second compensation point (xc) can be reached by substututing Mn(2a) with an early transition metal element X in Mn2YZ. In this case, we obtain a compensated ferrimagnet of a quarternary composition (e.g., Mn1.5V0.5FeAl). Due to a larger number of different atomic types in a unit cell, the system avoids the electronic insability which was present for Mn3Z composition and crystallizes in a cubic structure. Thus, the Mn-rich Heusler family provides two types of compensated ferrimagents - (i) ternary Mn3-yYyZ (Y - late transition element), which are tetragonal and exhibit magnetocrystalline anisotropy, and (ii) quarternary Mn2-xXxYZ (X - early transition element), which are cubic and are proximate to a half-metallic behavior.
In order to model more specific details in Heusler systems, such as the degree of the chemical order, or noncollinearity in the magnetic order, magnetocrystalline anisotropy, electronic transport properties and spectroscopies, the use of the first-principles computations is necessary. In the following we outline our selected resiults in the sections of magnetism and electronic transport.


